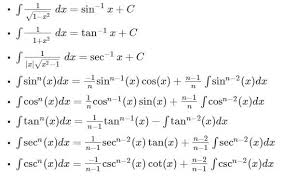You can download the Integration Formulas PDF for free by using the direct link provided below on the page.
Integration Formulas PDF
Integration, also known as inverse differentiation, is a mathematical process that can be considered as the reverse of differentiation. It involves finding a function whose derivative is a given function. In other words, integration allows us to determine the original function when only its derivative is known.
An integral is a mathematical function that represents the area under a curve or the accumulation of a quantity. By performing integration, we can calculate the areas of two-dimensional regions and compute volumes of three-dimensional objects. This makes integration a powerful tool in various fields, including physics, engineering, economics, and more.
One of the main applications of integration is in finding the area under a curve. This process, known as definite integration, involves evaluating the integral of a function within specific limits. By determining the area under a curve, we can analyze various phenomena, such as calculating the total distance traveled by an object or determining the total sales revenue over a specific time period.
Integration also plays a crucial role in determining the antiderivative of a function. The antiderivative, also called the indefinite integral, represents a family of functions that have the same derivative. By finding the antiderivative of a function, we can obtain a general solution that encompasses all possible functions with the same derivative.
Integration is used to solve differential equations, which are equations that involve derivatives. By integrating both sides of a differential equation, we can find the general solution that satisfies the equation. This allows us to model and understand various physical phenomena, such as population growth, fluid flow, and electrical circuits. Integration is a mathematical process that involves finding a function with its derivative.
It is the reverse of differentiation and allows us to determine the original function when only its derivative is known. Integration is used to calculate areas of two-dimensional regions, compute volumes of three-dimensional objects, solve differential equations, and find antiderivatives. Its applications extend to various fields, making integration a fundamental concept in mathematics and its practical applications.